a)

b)

a)
b)

a)
b)

a)
b)

0)

10000CE403122001

100 kN
‏جو‎
‎50k ~~ _
8 28 ८
3
30 8 ‏اع‎ =

A

Derive the relationship between force transformation matrix and displacement
transformation matrix.

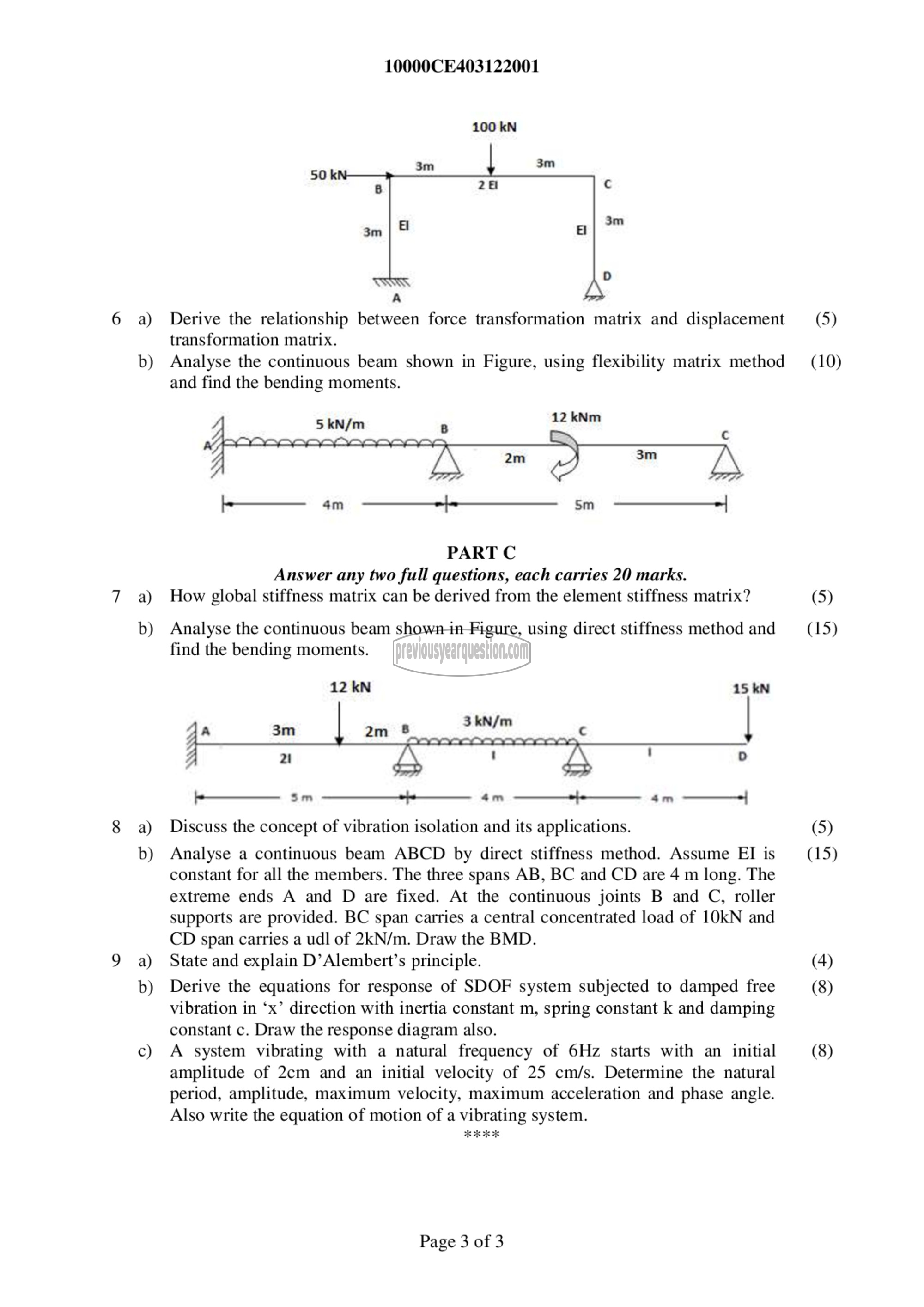
Analyse the continuous beam shown in Figure, using flexibility matrix method
and find the bending moments.

ത ത്ത ज kN/m 5 12 kNm ८
r= oA

ಕದ್ದು का -"{-- 5m ‏طلسن ل كك‎

PART C
Answer any two full questions, each carries 20 marks.
How global stiffness matrix can be derived from the element stiffness matrix?

Analyse the continuous beam shown in Figure, using direct stiffness method and
find the bending moments.

12 kN 15 kN

‎ee नी‏ _ مم

‎Discuss the concept of vibration isolation and its applications.

‎Analyse a continuous beam ABCD by direct stiffness method. Assume EI is
constant for all the members. The three spans AB, BC and CD are 4 m long. The
extreme ends A and D are fixed. At the continuous joints B and C, roller
supports are provided. BC span carries a central concentrated load of 1010 and
CD span carries a पत्‌] of 2kN/m. Draw the BMD.

‎State and explain D’Alembert’s principle.

‎Derive the equations for response of SDOF system subjected to damped free
vibration in ‘x’ direction with inertia constant m, spring constant k and damping
constant c. Draw the response diagram also.

‎A system vibrating with a natural frequency of 6Hz starts with an initial
amplitude of 2cm and an initial velocity of 25 cm/s. Determine the natural
period, amplitude, maximum velocity, maximum acceleration and phase angle.

‎Also write the equation of motion of a vibrating system.
मं मर मर मर

‎Page 3 of 3

‎(5)

‎(10)

‎(5)
‎(15)

‎(5)
‎(15)

‎(4)
‎(8)

‎(8)