12 a)

b)

13

14

15

16

17 ஐ
b)

F192030 Pages:2

PART C
Answer any two full questions, each carries 10 marks.

A system is described by x(t) = 9 A x(t)

Determine state transition matrix for the system.

Define controllability. Explain with a suitable example, how can we check the
controllability of a system?

Derive the state model of the following transfer function in,

(i) Controllable canonical form
(11) Diagonal canonical form
(5) _ 5(5 +2)

(5) 5(5 + 1) (5 + 3)
Examine the stability of the system with the following characteristic equation
using Jury’s stability test.
21 — 1.25 + 0.0722 + 0.32 - 0.08 = 0

PART D
Answer any two full questions, each carries 10 marks.
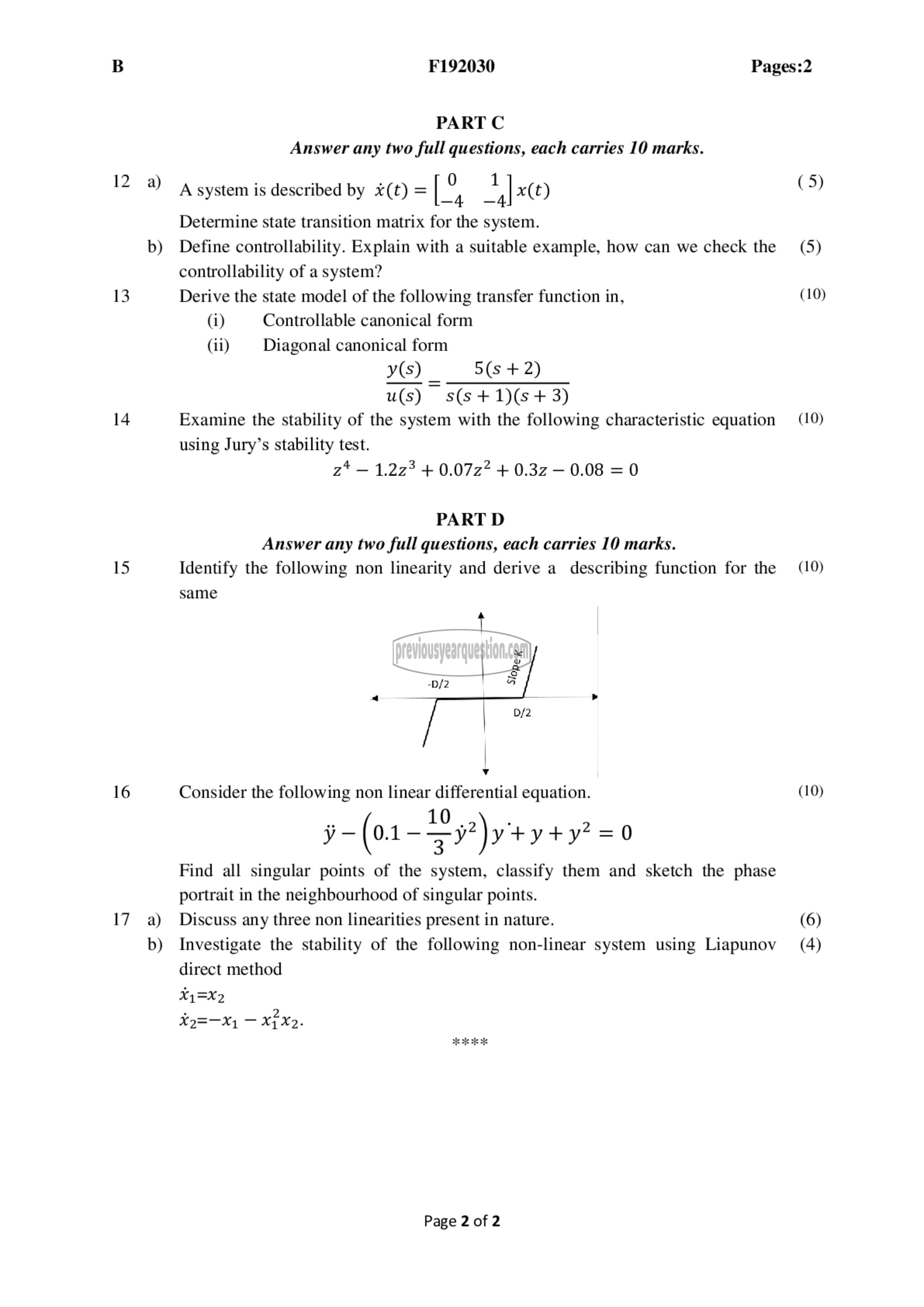
Identify the following non linearity and derive a describing function for the
same

[ग

Consider the following non linear differential equation.

8 10. ⋅
⊃∣−≺∘⋅↥−∍−⊃↗∄≻⊃∣⊹⊃∣⊹⊃↗≳ =0

Find all singular points of the system, classify them and sketch the phase
portrait in the neighbourhood of singular points.

Discuss any three non linearities present in nature.

Investigate the stability of the following non-linear system using Liapunov
direct method

X4=X2

X2=—-X14 - x2 x2.

بد بد ‎ಸೇರ‏

Page 2 of 2

(5)

(5)

(10)

(10)

(10)

(10)

(6)
(4)