നതി
601
2
8 a. Consider the discrete timesystem defined by the equation (6)
௦ ० -0.25 0 0 1
x(k+|)= Hu(k)whereG= ௦ 1 0 016 = .11-
[1 00] . .y(k) = cx(k) 0 1 ]

Assuming that the output y{k) is measurable, design a minimum order observer, such
that the error will exhibit deadbeat response.

0. Prove that if a discrete system is completely state controllable and observable, (6) then
there is no pole zero cancellation in the pulse transfer function.
(6 marks)
9a. Consider a multi output linear system described by the state model (4)

x(k+1)= Fx(k) +Gu(k) y(k)
= cx(k) - Du(k)
0

! 8 9 1 0 1 1 0
=| 0 0 ١)6 ‏ا6اہ 1ع‎ | ۳ ം |
-2 1 4] 1 1

where, F
Check whether the system is observable.

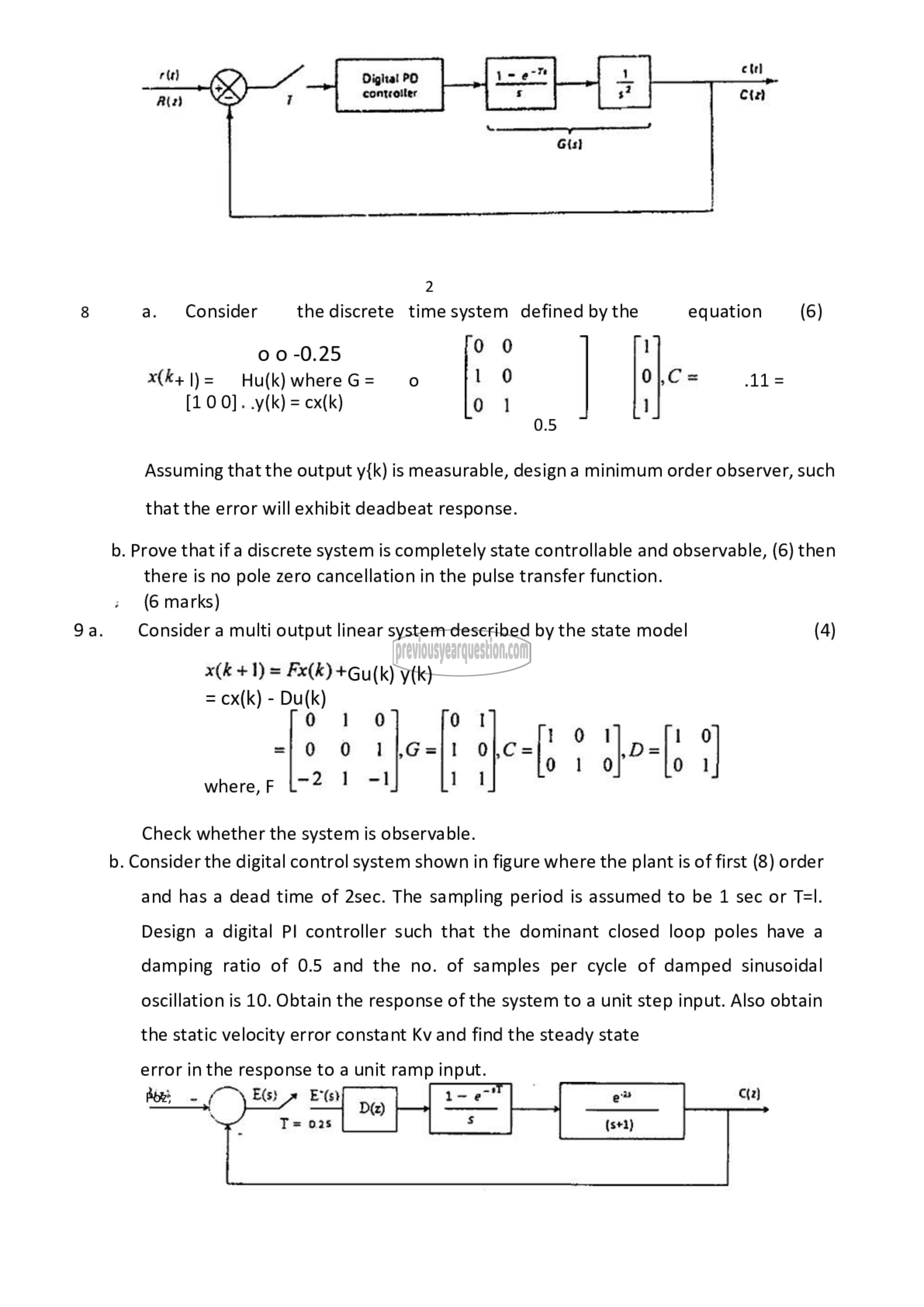
b. Consider the digital control system shown in figure where the plant is of first (8) order
and has a dead time of 2sec. The sampling period is assumed to be 1 sec or 151.
Design a digital Pl controller such that the dominant closed loop poles have a
damping ratio of 0.5 and the no. of samples per cycle of damped sinusoidal
oscillation is 10. Obtain the response of the system to a unit step input. Also obtain
the static velocity error constant Kv and find the steady state

error inthe response to a unit ramp input.