12

13

14

15

16

17

a)

b)

B5811 Pages: 2

G(s) = ಷ್‌ .Determine the gain K so that the system will have a damping

ratio of 0.5
PART C
Answer any two full questions, each carries 10 marks
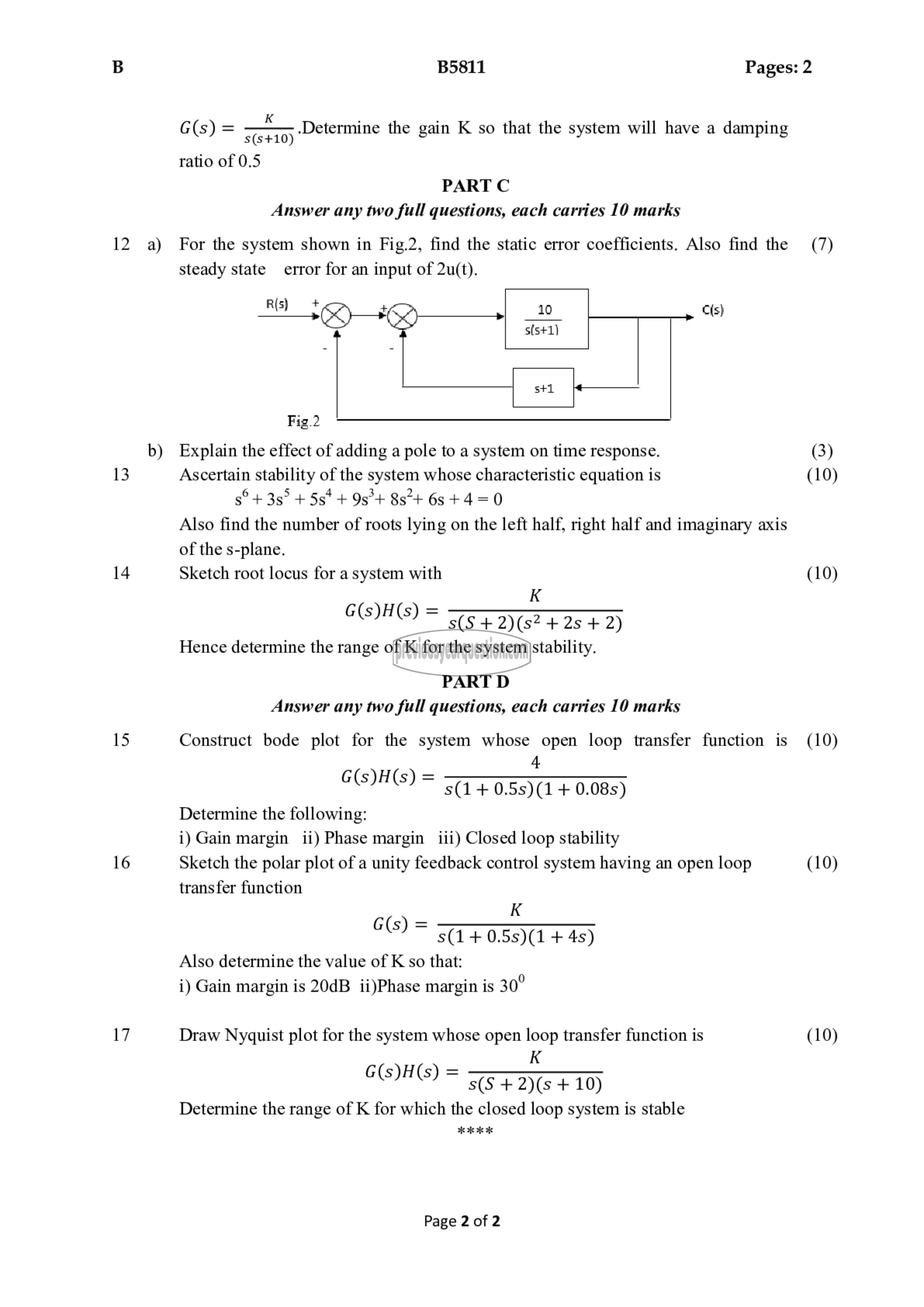
For the system shown in Fig.2, find the static error coefficients. Also find the
steady state error for an input of 2u(t).

R(s) eK) +, >| 10 55 05)

5(5+1]

5+1 ಶ್ಚ ‏خخ‎

71 2
Explain the effect of adding a pole to a system on time response.
Ascertain stability of the system whose characteristic equation is
s°+3s° + 554 + 9s*+ ‏+خوع‎ 65 + 4 = 0
Also find the number of roots lying on the left half, right half and imaginary axis
of the s-plane.
Sketch root locus for a system with
K
s(S + 2)(s? + 25 + 2)
Hence determine the range of K for the system stability.
PART D
Answer any two full questions, each carries 10 marks

G(s)H(s) =

Construct bode plot for the system whose open loop transfer function is
4
6070) = उत ~न तफ 0.085)

Determine the following:
i) Gain margin ii) Phase margin iii) Closed loop stability
Sketch the polar plot of a unity feedback control system having an open loop
transfer function

K
5(1 + 0.5s)(1 + 45)
Also determine the value of K so that:
i) Gain margin is 20dB ii)Phase margin is 30°

G(s) =

Draw Nyquist plot for the system whose open loop transfer function is
K

s(S + 2)(s + 10)

Determine the range of K for which the closed loop system is stable
‏یاد ید‎ KK

G(s)H(s) =

Page 2 of 2

(7)

(3)
(10)

(10)

(10)

(10)

(10)