B D192019 Pages:2

Reg No.: Name:

APJ ABDUL KALAM TECHNOLOGICAL UNIVERSITY
FOURTH SEMESTER B.TECH DEGREE EXAMINATION(S), DECEMBER 2019
Course Code: EC202
Course Name: SIGNALS & SYSTEMS
Max. Marks: 100 Duration: 3 Hours
PARTA
Answer any two full questions, each carries 15 marks. Marks

1 a) Check whether the following signals are periodic or not. If periodic, find the fundamental (8)

period. (i) x(t) = sin(200zxt) + cos(150xt) (1) x[n] = sin(0.15zn) + 008(0. 477)
b) Check whether the system, y(t) = ×00 is (7)
(i) Linear (ii) Time-Invariant (iii) Causal (1४) Stable.

{+ 1-1 > > 0 )12(

Given x(t) = ಗ O0 ;otherwise

Find y(t) = x(t) * h(t); where "*' denotes convolution. Also plot x(t), h(t) and y(t)

b) Check the causality and stability of the LTI system with impulse response (3)
A(t) = e7?*u(t + 2)
3 a) Given x(t) = u(t+1) + u(t-1) - u(t-2) —u(t-4). (8)

Plot (i)x() (11) ೫(1-3) (൨2൧2൧) (iv) x(2t-3)
0) What is the condition for two signals x(t) and y(t) to be orthogonal? Give example of two (3)
signals which are orthogonal.
€) Show that the output of an LTI system with impulse response 11/11 to the input x/n] is the (4)
convolution sum of x/n] and h[n].
PART B
Answer any two full questions, each carries 15 marks.
4 a) State the conditions for convergence of Fourier Series. Also give an example (with (9)
waveform) each, for the signals that does not satisfy the conditions.

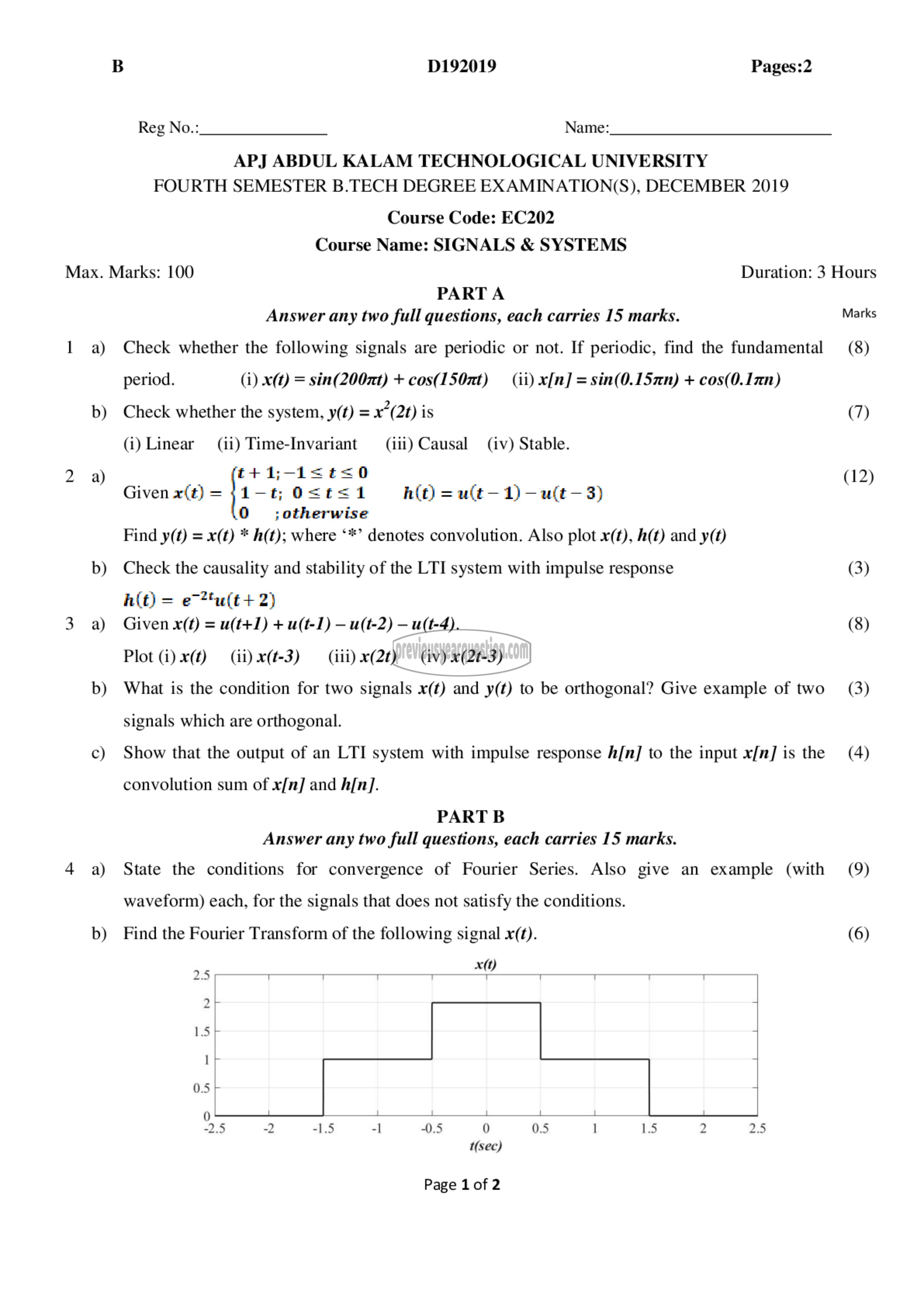
b) Find the Fourier Transform of the following signal x(t). (6)

xi)

25 === 1

t(sec)

Page 1 of 2