B B4812 Pages: 3

PART B
Answer any two questions, each carries 15 marks
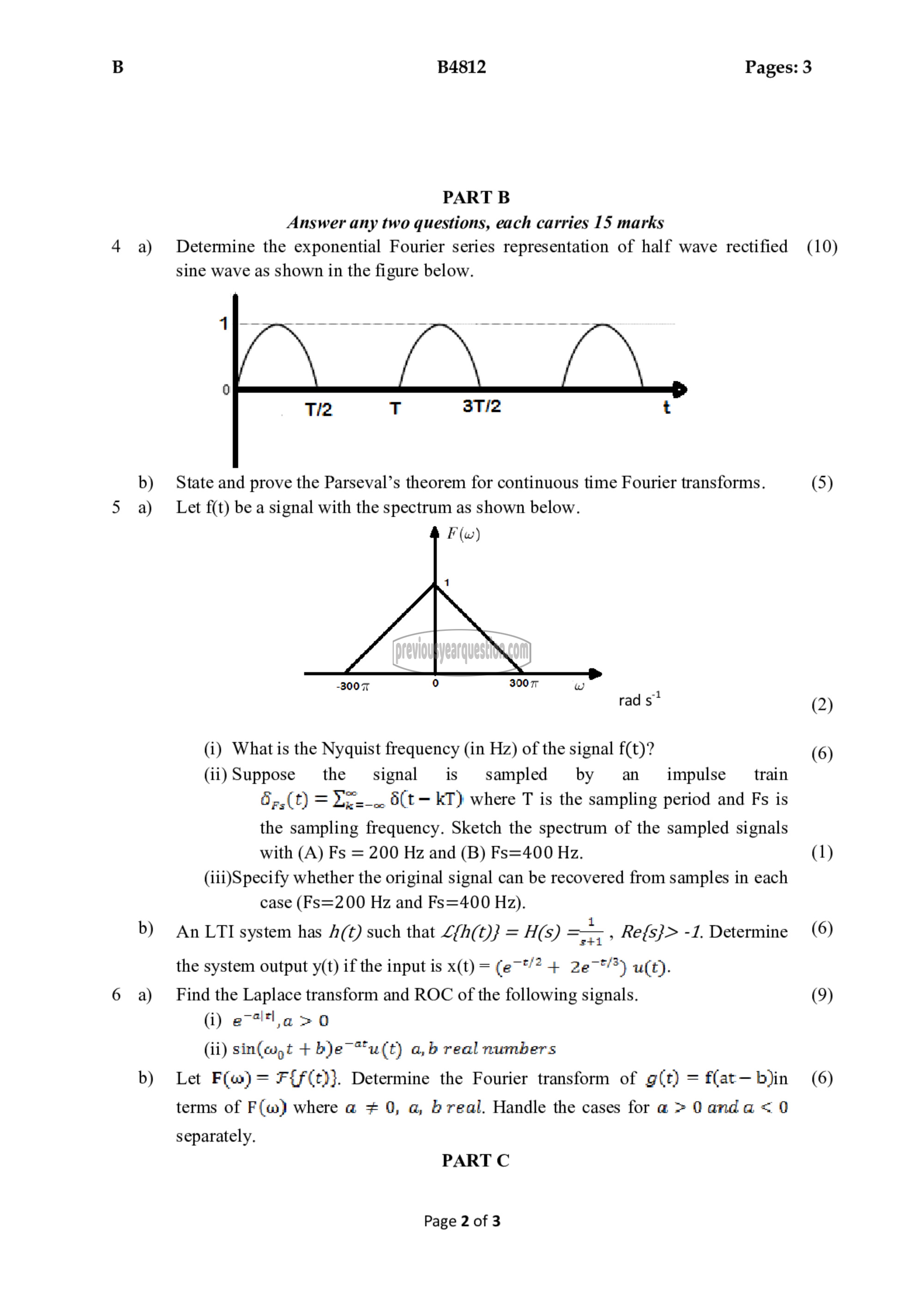
4 a) Determine the exponential Fourier series representation of half wave rectified (10)
sine wave as shown in the figure below.

b) State and prove the Parseval’s theorem for continuous time Fourier transforms. (5)
5 9) Let f(t) be a signal with the spectrum as shown below.

-3007 0 3007 ய

rad 57 (2)

(i) What is the Nyquist frequency (in Hz) of the signal f(t)? (6)
(ii)Suppose the signal is sampled by ஹூ impulse _ train

5,,(t) = 2. 6(t — kT) where T is the sampling period and Fs is

the sampling frequency. Sketch the spectrum of the sampled signals

with (A) Fs = 200 Hz and (B) Fs=400 Hz. (1)
(iii)Specify whether the original signal can be recovered from samples in each

case (Fs=200 Hz and Fs=400 Hz).

b) An LTI system has A(t) such that ൧൧൫൦ = H(s) = , Re{s}>-1. Determine (6)
the system output y(t) if the input is x(t) = (e~*/? 2௪515) u(t).
6 2) Find the Laplace transform and ROC of the following signals. (9)
(1) ௪31 ௨0
(11) sin(wa,t മുട ഡ്‌) 6, 9 real numbers
b) Let F(w)= F{fF(t)}. Determine the Fourier transform of g(t) =f(at—b)in (6)
terms of F(w) where a = 0, a, b real. Handle the cases for a > Qanda > 0

separately.
PART C

Page 2 of 3