b)

a)

b)

a)

b)

a)

b)

G192147 Pages:3

Write the dual of the following LPP:

Mini z = 3x, +4x>+5x;

Subject to:

X)+2x7t+4x3>12

2x)+x2+5x3 = 5

430) +7x2+6x3<8

20/32 20 ஐடி is unrestricted in sign

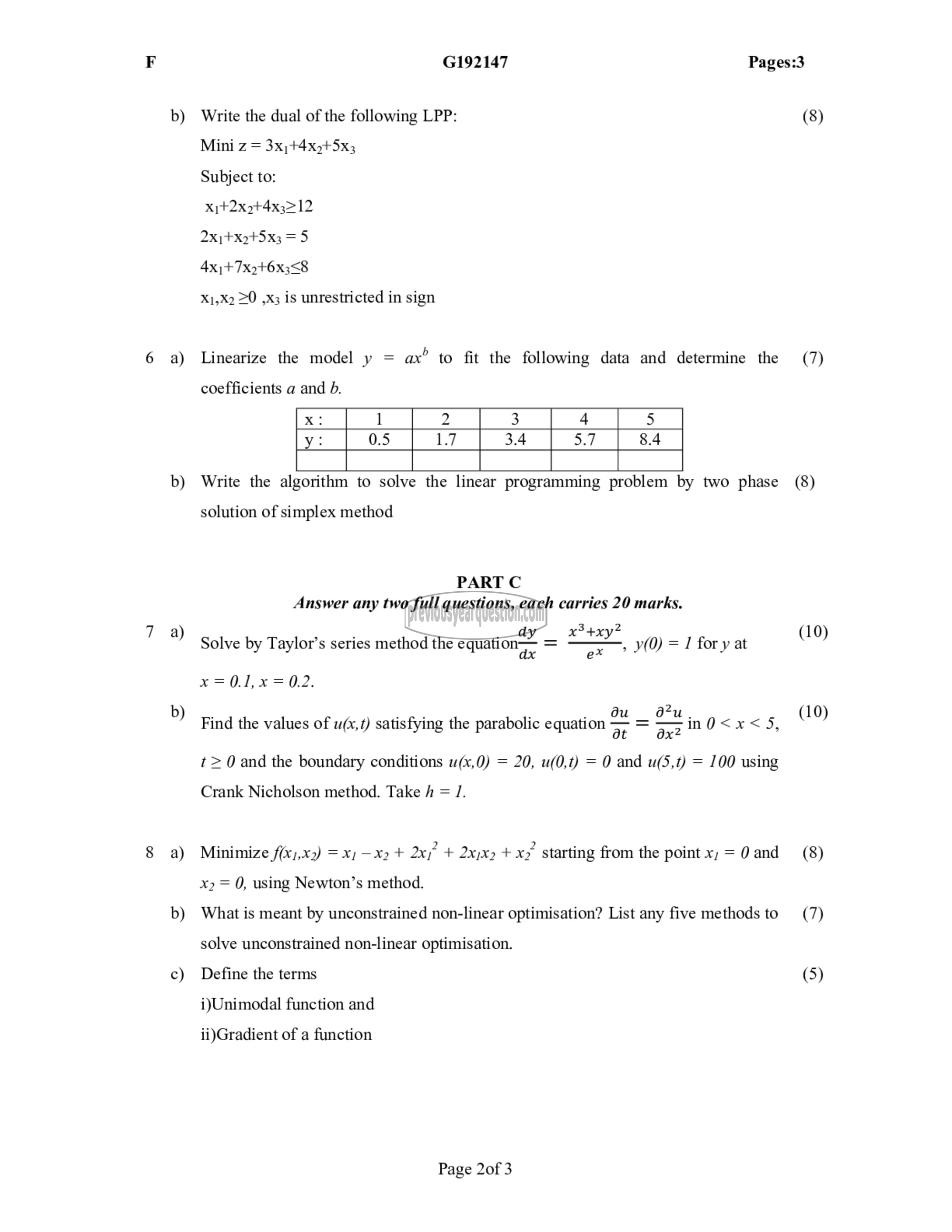
Linearize the model ‏بر‎ = ax’ to fit the following data and determine the

coefficients a and b.

Write the algorithm to solve the linear programming problem by two phase

solution of simplex method

PART C
Answer any two full questions, each carries 20 marks.

८3 + ‏ن0‎
‎ex

d
Solve by Taylor’s series method the equation = + ¥(0) = 1 for y at

x =0.1,x = 0.2.

⋅⇊⊋∂↓↧∂⋅ ⋅ ⋅∙ 5
و3 > ع > 0 ل 77 ⋂∘∏⊟⋯⊏⊜∁∐∘↻⊞∂⊃⇂⊜↿∤↥≣⋯∣⋝∱⊰⋯≌⊱∩∣∝∣∥∌∘⊰⊜∐∣∅⋁⊜↧∤↥↿≺⋯∶⇂

t > 0 and the boundary conditions u(x,0) = 20, u(0,)) = 0 and u(5,t) = 100 using
Crank Nicholson method. Take h = 7.

Minimize f(x),x2) = x); —x2 + മഗ്‌ + 2x)x2 + xy starting from the point x; = 0 and
‏جد‎ = 0, using Newton’s method.

What is meant by unconstrained non-linear optimisation? List any five methods to
solve unconstrained non-linear optimisation.

Define the terms

i)Unimodal function and

ii)Gradient of a function

Page 2of 3

(8)

(7)

(8)

(10)

(10)

(8)

(7)

(5)