b)
a)

b)

(c)

a)

b)

a)

b)

९)

2)

b)

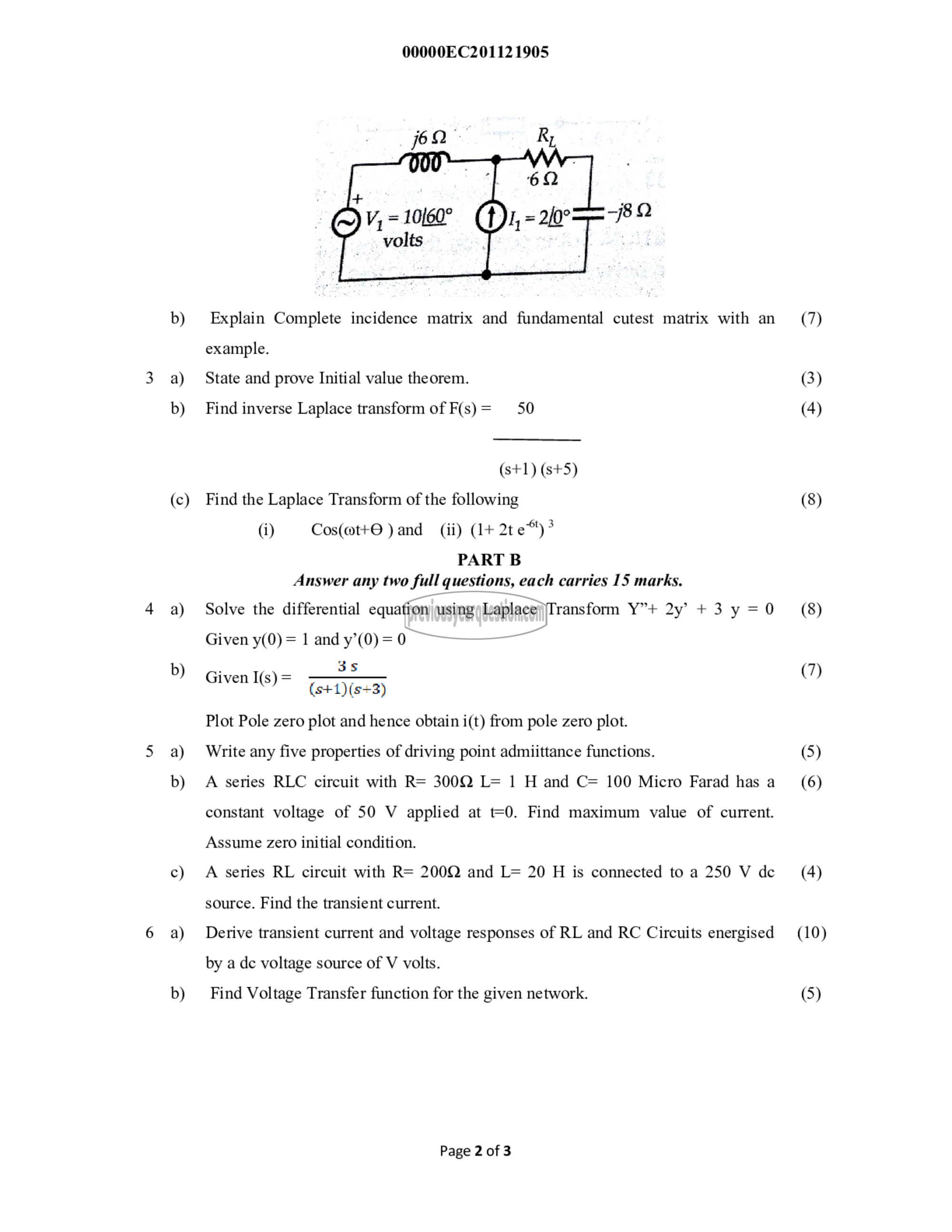
00000EC201121905

Explain Complete incidence matrix and fundamental cutest matrix with an
example.
State and prove Initial value theorem.

Find inverse Laplace transform of F(s)= 50

(st1) (815)
Find the Laplace Transform of the following
(i) ‏ڈكدہ‎ م۳٥‎ ) and (1) (1+ 2t €) 3

PART ‏تا‎
‎Answer any two full questions, each carries 15 marks.

Solve the differential equation using Laplace Transform Y”+ 2y’ + 3 y ८०
Given y(0) = 1 and y’(0)=0

Given I(s) = ணை
Plot Pole zero plot and hence obtain i(t) from pole zero plot.

Write any five properties of driving point admiittance functions.

A series RLC circuit with R= 300Q L= 1 H and C= 100 Micro Farad has a
constant voltage of 50 V applied at t=0. Find maximum value of current.
Assume zero initial condition.

A series RL circuit with R= 200Q and L= 20 H is connected to a 250 V de
source. Find the transient current.

Derive transient current and voltage responses of RL and RC Circuits energised

by a de voltage source of V volts.

Find Voltage Transfer function for the given network.

Page 2 of 3

(7)
(3)

(4)

(8)

(8)

(7)

(5)

(6)

(4)

(10)

(5)