b)

3 a)

b)

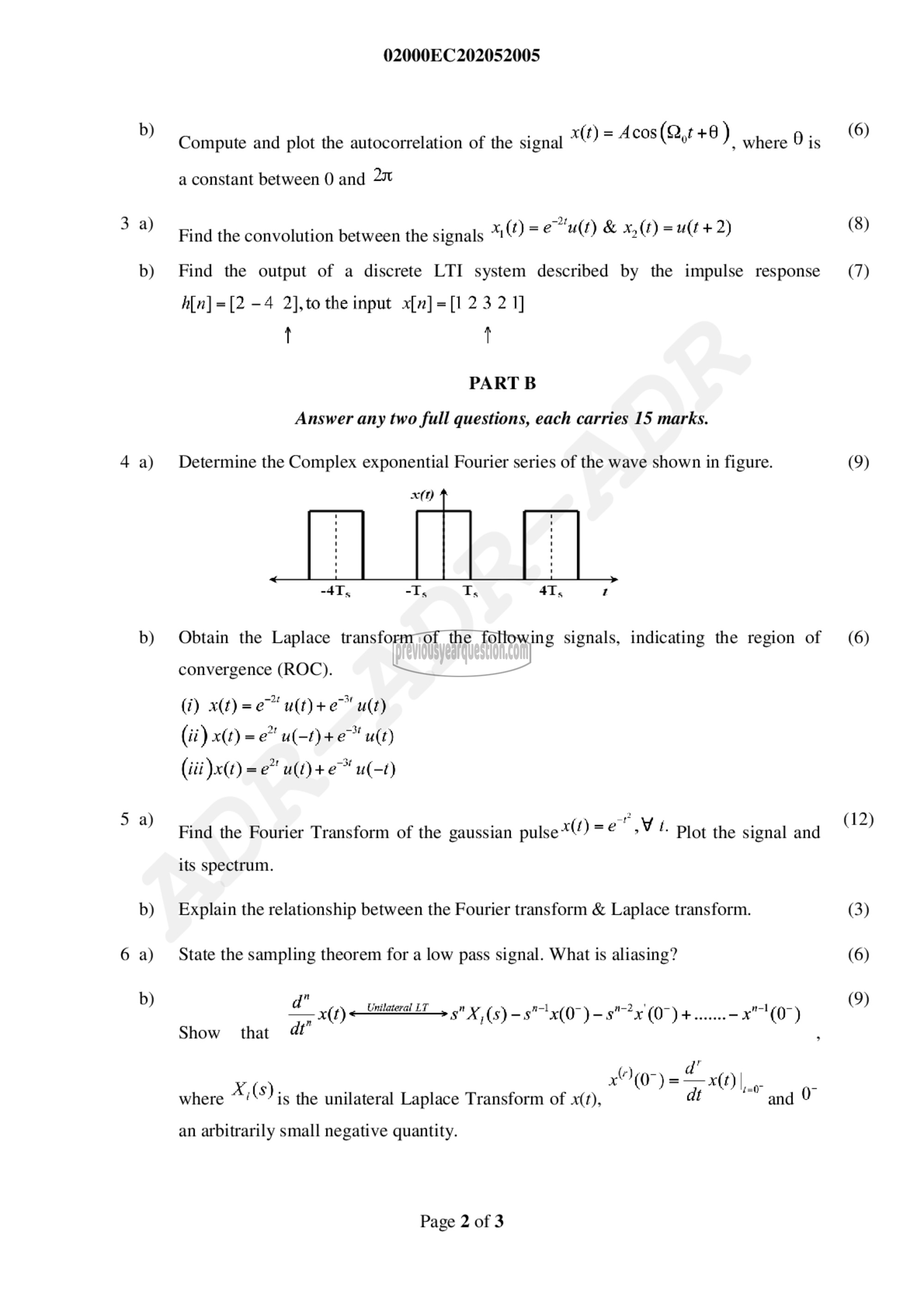
4 a)

b)

5 a)

b)
6 a)

b)

02000EC202052005

Compute and plot the autocorrelation of the signal

a constant between 0 and 2%

Find the convolution between the signals

Find the output of a discrete LTI system described by the impulse response

h[n] = [2 -4 2],to the input प] = [12321]
t 1

PART ‏تا‎

x(t) = Acos(Q,t +8 )

x,(t) = e“u(t) & x,(t) = u(t + 2)

Answer any two full questions, each carries 15 marks.

Determine the Complex exponential Fourier series of the

x(t)

-4T, ഇ ஆ 47, ൧

Obtain the Laplace transform of the following signals, indicating the region of

convergence (ROC).

(i) x(t) =e" u(t) +1 u(t)
(ii) x(t) = ४ ४(-/)+ € u(t)
(111)(1) =e" u(t) +< * ४(-/)

Find the Fourier Transform of the gaussian pulse *(/) =<" Wi. Plot the signal and

its spectrum.
Explain the relationship between the Fourier transform &

State the sampling theorem for a low pass signal. What is

Show that 4”

X;,(s);

where is the unilateral Laplace Transform of ൧൧,

an arbitrarily small negative quantity.

Page 2 of 3

wave shown in figure.

Laplace transform.

aliasing?

4 x(t) அவம்‌ > 5" (5) - 5" (0 )- 5" 2x (0) +... x" (0)

(८) 0 ച്‌ (7
mo) न

, where Dis

५०

(6)

(8)

(7)

(9)

(6)

(12)

(3)
(6)
(9)