a. Derive the stiffness matric for a CST element using the principle of minimization of
potential energy. 7
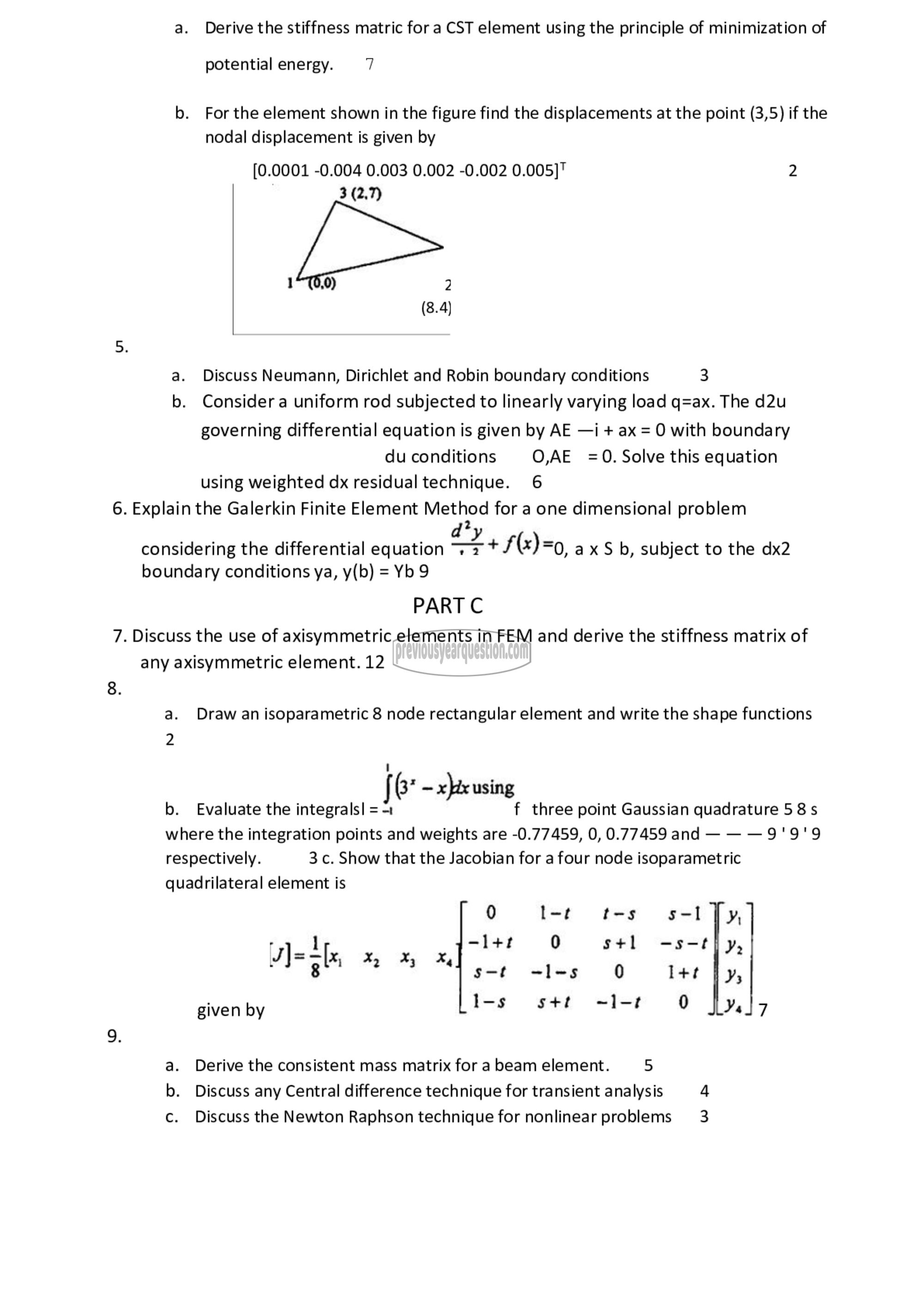
0. For the element shown in the figure find the displacements at the point (3,5) if the

nodal displacement is given by

[0.0001 -0.004 0.003 0.002 -0.002 0.005]7 2
3 (2.7)

1 [> 2
(8.4)

a. Discuss Neumann, Dirichlet and Robin boundary conditions 3
Consider 8 uniform rod subjected to linearly varying load q=ax. The d2u
governing differential equation is given by AE —i + ax = 0 with boundary
du conditions O,AE ಎ0. Solve this equation
using weighted dx residual technique. 6
6. Explain the Galerkin Finite Element Method for a one dimensional problem

43)
considering the differential equation ‏جب‎ + f(x) “0, ax ऽ 0, subject to the 22
boundary conditions ya, y(b) = Yb 9
PART C

7. Discuss the use of axisymmetric elements in FEM and derive the stiffness matrix of
any axisymmetric element. 12

8.
a. Draw an isoparametric 8 node rectangular element and write the shape functions
1
[8 ~x}x using
0. Evaluate the integrals! = -1 ர்‌ three point Gaussian quadrature 5 8 5
where the integration points and weights are -0.77459, 0, 0.77459 and — — -- 9 '9' 9
respectively. 3 ©. Show that the Jacobian for a four node isoparametric
quadrilateral element is
0 1! 1-3 தல] |)
1 -l+t 0 851 தர்‌
ಗ ೫; ೩, ಹ್ಮ | 0 1 2
t -l-s +t Ty,
given by 1-8 553.1 -1-( 0 ‏ول[‎ 7
9.
a. Derive the consistent mass matrix for a beam element. 5

b. Discuss any Central difference technique for transient analysis 4
Discuss the Newton Raphson technique for nonlinear problems 3