b) An air compressor of 500*kg operates at a constant speed of 1 750 rev/min. Rotating parts are
well balanced. The reciprocating part is 10 kg and crank radius is 100 mm. The mounting
introduces an effective damping of damping factor 0.15- Specify the spring for the mounting such
that only 18% of the unbalanced force is transmitted to the foundation. Also determine the
amplitude of transmitted force

(5 marks)

Part B

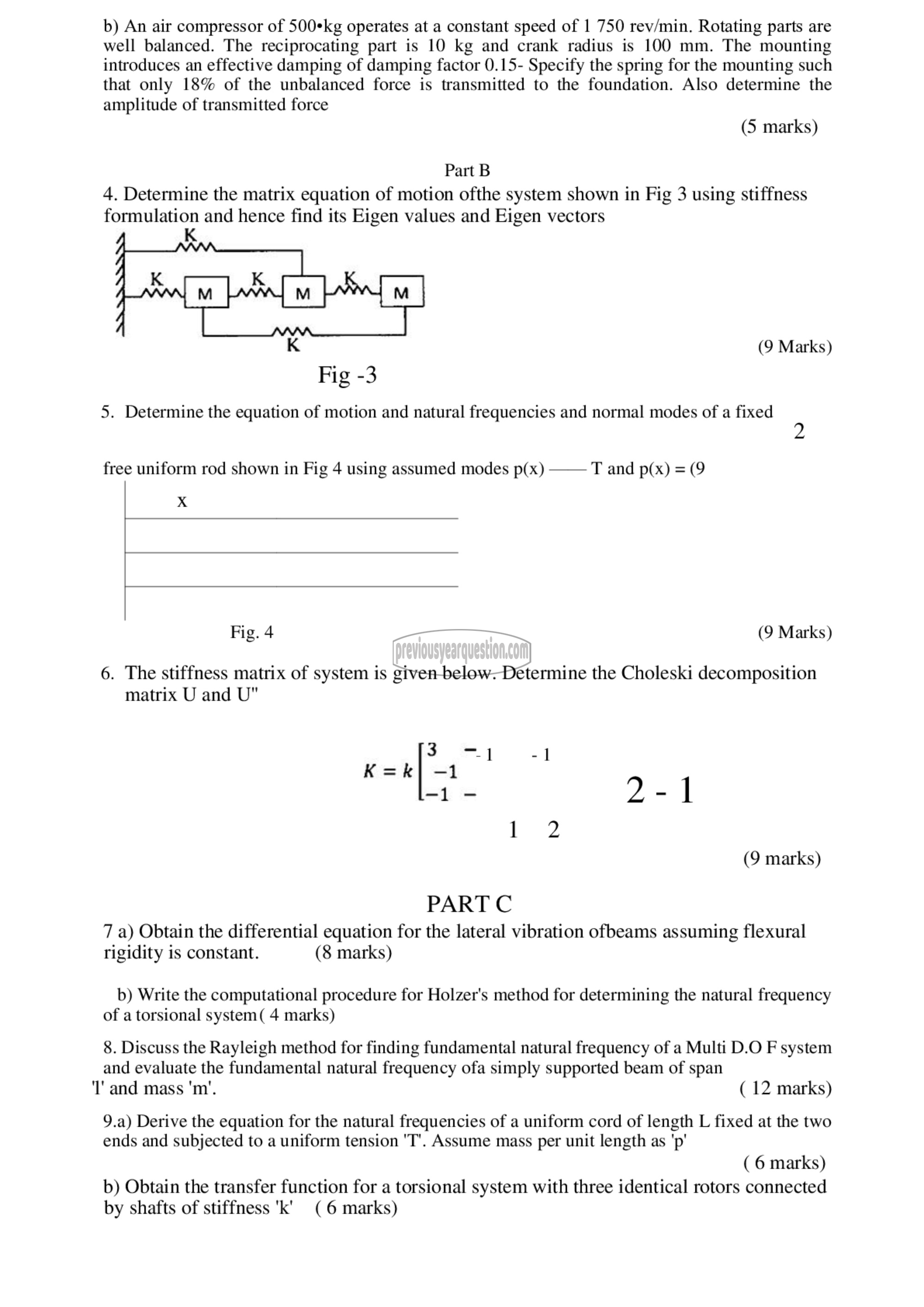
4. Determine the matrix equation of motion ofthe system shown in Fig 3 using stiffness

formulation and hence find its Eigen values and Eigen vectors
K

(9 Marks)
Fig -3
5. Determine the equation of motion and natural frequencies and normal modes of a fixed

2

free uniform rod shown in Fig 4 using assumed modes p(x)

T and p(x) = 9
18...

Fig. 4 (9 Marks)

6. The stiffness matrix of system is given below. Determine the Choleski decomposition
matrix U and U"

(9 marks)

PART (^
7 a) Obtain the differential equation for the lateral vibration ofbeams assuming flexural
rigidity is constant. (8 marks)

b) Write the computational procedure for Holzer's method for determining the natural frequency
of a torsional system( 4 marks)

8. Discuss the Rayleigh method for finding fundamental natural frequency of a Multi D.O F system
and evaluate the fundamental natural frequency ofa simply supported beam of span
'1' and mass ൬൩. ( 12 marks)

9.a) Derive the equation for the natural frequencies of a uniform cord of length L fixed at the two
ends and subjected to a uniform tension 'T'. Assume mass per unit length as 'p'

( 6 marks)
b) Obtain the transfer function for a torsional system with three identical rotors connected
by shafts of stiffness 'k' (6 marks)