10

11

12

13

14

B7075

Derive from fundamentals pure bending equation = =. Also state the (10) important
assumptions.

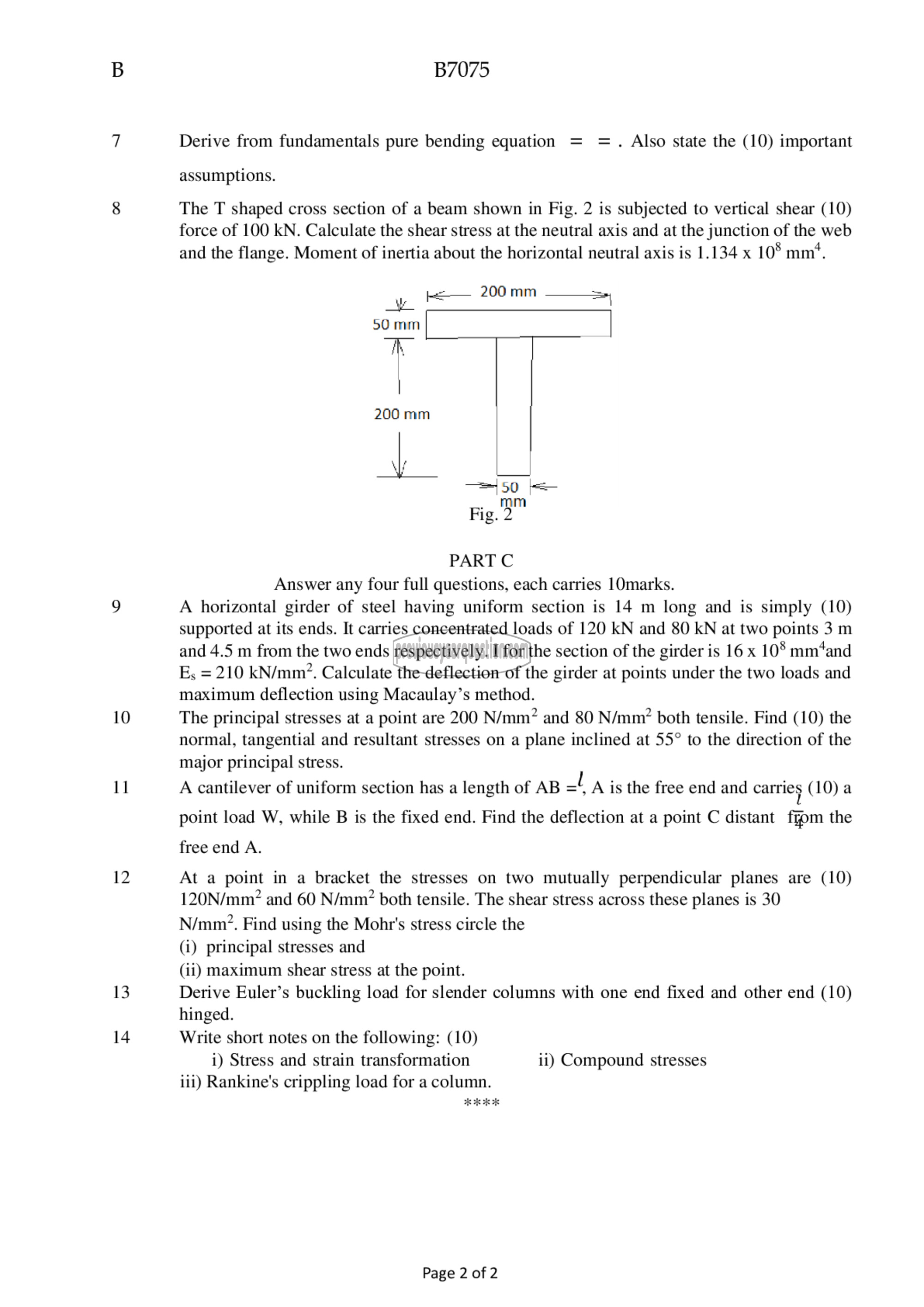
The T shaped cross section of a beam shown in Fig. 2 is subjected to vertical shear (10)
force of 100 KN. Calculate the shear stress at the neutral axis and at the junction of the web

and the flange. Moment of inertia about the horizontal neutral axis 15 1.134 x 107 mm‘.

‎ಆ. 200mm ದಾ‏ رف
‎೨೦ mm‏

‎ரிட்‌

‎|
‎200 mn

‎+ ||

‎அவி ‏ےا ںو‎
Fi mm
ig. 2

‎PART ^
Answer any four full questions, each carries 10marks.

‎A horizontal girder of steel having uniform section is 14 m long and is simply (10)
supported at its ends. It carries concentrated loads of 120 kN and 80 KN at two points 3 m
and 4.5 m from the two ends respectively. I for the section of the girder is 16 x 108 mm‘and
‏وط‎ = 210 kN/mm*. Calculate the deflection of the girder at points under the two loads and
maximum deflection using Macaulay’s method.

‎The principal stresses at a point are 200 N/mm? and 80 N/mm’ both tensile. Find (10) the
normal, tangential and resultant stresses on a plane inclined at 55° to the direction of the
major principal stress.

‎A cantilever of uniform section has a length of AB _! A is the free end and carries (10) 8
point load W, while B is the fixed end. Find the deflection at a point C distant fom the
free end A.

‎At a point in a bracket the stresses on two mutually perpendicular planes are (10)
120N/mm/? and 60 N/mm? both tensile. The shear stress across these planes is 30
N/mm”. Find using the Mohr's stress circle the
(i) principal stresses and
(ii) maximum shear stress at the point.
Derive Euler’s buckling load for slender columns with one end fixed and other end (10)
hinged.
Write short notes on the following: (10)
i) Stress and strain transformation ii) Compound stresses
iii) Rankine's crippling load for a column.

‎Page 2 of 2