(6)

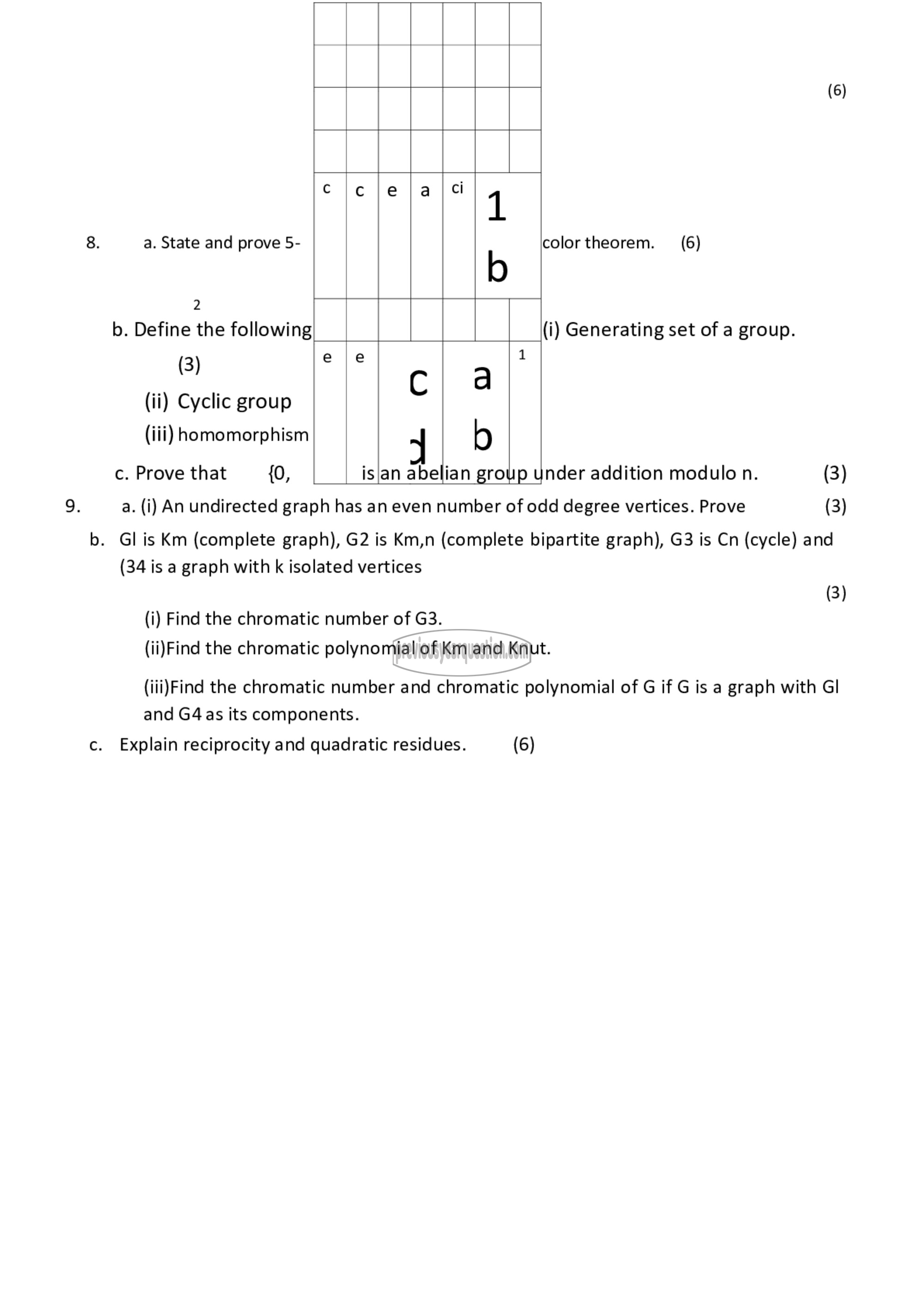
8. a. State and prove 5- b colortheorem. (6)
2
b. Define the following (i) Generating set of a group.
1
(3) ١ 1 3
(ii) Cyclic group ட
(iii) homomorphism b
c. Prove that 10, 153೧ abelian group under addition modulo 0. (3)
9. a. (i) An undirected graph has an even number of odd degree vertices. Prove (3)

0. Gl is Km (complete graph), G2 is Km,n (complete bipartite graph), G3 is Cn (cycle) and
(34 is a graph with k isolated vertices
(3)
(i) Find the chromatic number of G3.
(ii)Find the chromatic polynomial of Km and Knut.

(iii)Find the chromatic number and chromatic polynomial of © if G is a graph with Gl
and G4as its components.

c. Explain reciprocity and quadratic residues. (6)