ii) P[-4
c. Define the following for a sequence of random variables 4
(i) Almost sure convergence
(ii) | Convergence in probability
(iii) | Convergence in Mean-square sense

(iv) | Convergence in distribution

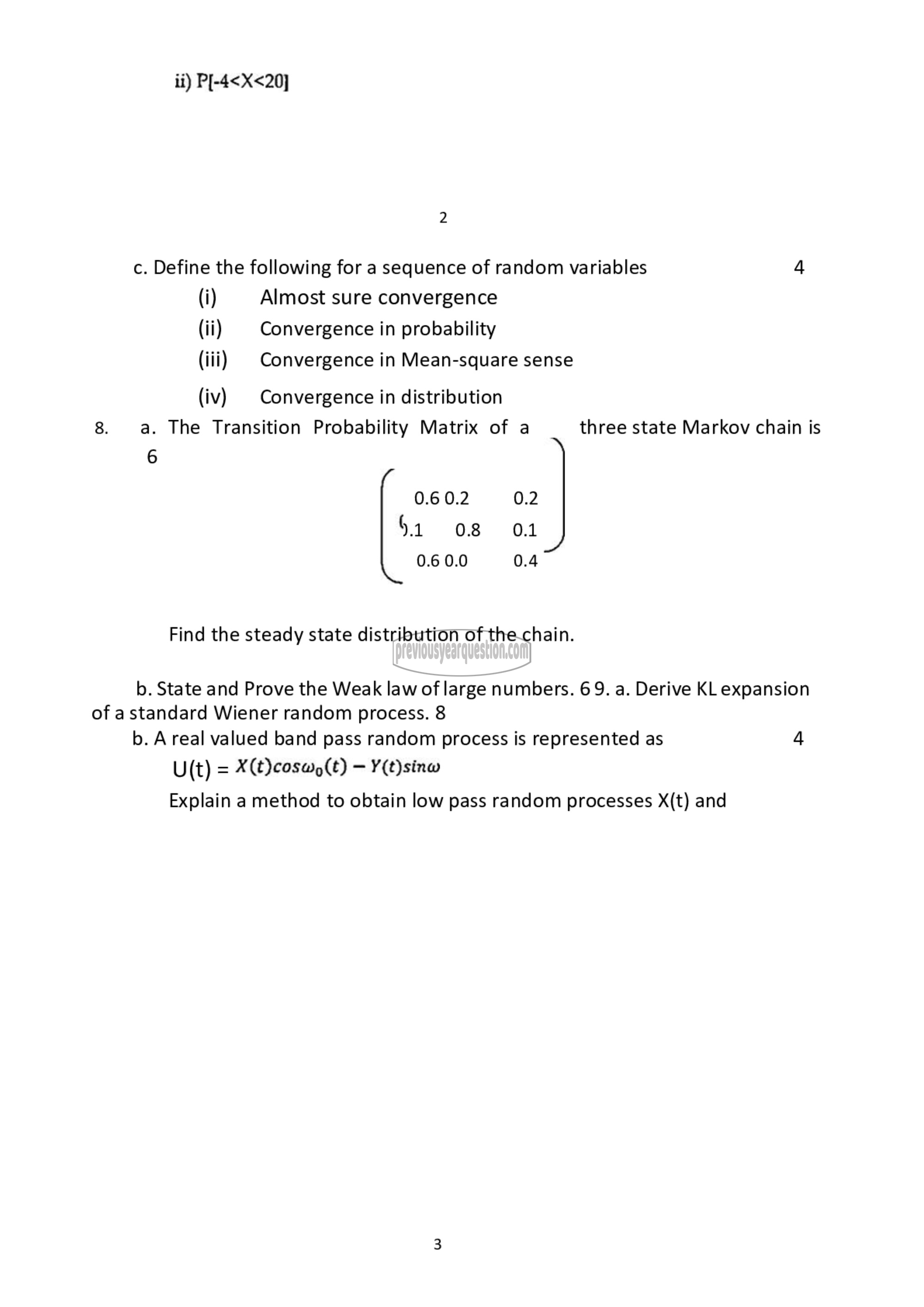
8. 9. The Transition Probability Matrix of a three state Markov chain is
6
0.6 0.2 0.2
५ 08 0.41
0.6 0.0 0.4

Find the steady state distribution of the chain.

b. State and Prove the Weak law of large numbers. 6 9. a. Derive KL expansion
of a standard Wiener random process. 8
b. Areal valued band pass random process is represented as 4
U(t) = * (1)208ம0(6) ~ നമ്മ

Explain a method to obtain low pass random processes X(t) and