1

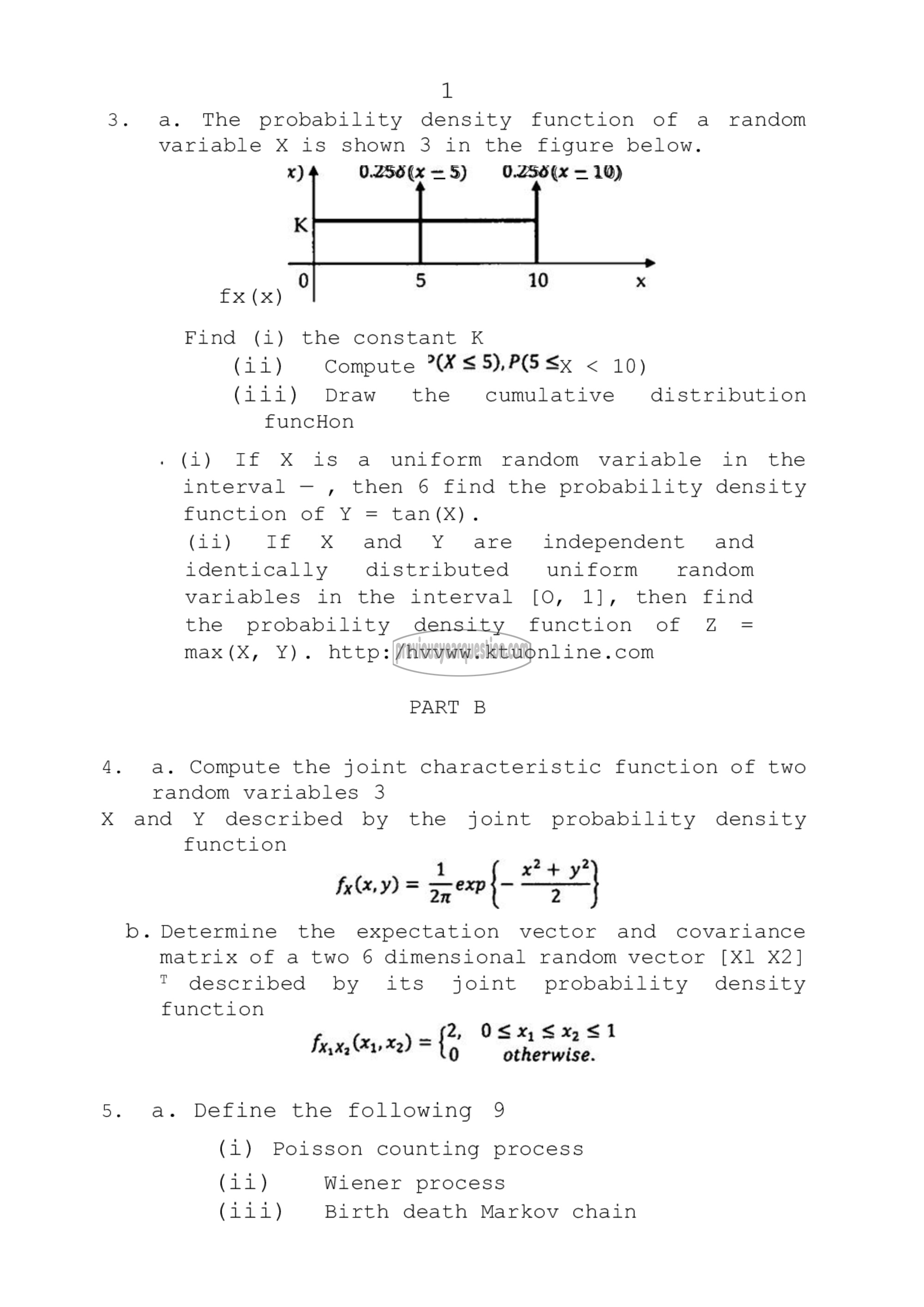
3. a. The probability density function of a random
variable X is shown 3 in the figure below.
ட. 0.252(2--5) 0०.25९ (८ - 10)

Find (i) the constant K
(11) Compute (४ € 5(,2)5 Sx < 10)

(111) Draw the cumulative distribution

funcHon

(1) If X is a uniform random variable in the
interval — , then 6 find the probability density

function of Y = tan(X).

(11) If ॐ and Y are independent and
identically distributed uniform random
variables in the interval [0, 1], then find

the probability density function of 2
max(X, Y). http:/hvvww.ktuonline.com

PART B

4. a. Compute the joint characteristic function of two

random variables 3

X and Y described by the joint probability density

function
1 ‏شير‎ y?
ட்டே) = तद्य {- ‏بہت‎

b. Determine the expectation vector and covariance
matrix of a two 6 dimensional random vector [Xl 20)
7 described by its joint probability density

function
ட ‏و2‎ 0 < x, < X2 <1
11203, = bo otherwise.

5. a. Define the following 9
(1) Poisson counting process

(11) Wiener process
(111) Birth death Markov chain