b) The forward transfer function of a unity feedback system is given as

K

G(s) = s(s + 3)(s + 6) . Design a lag-lead compensator so that the system

satisfies the following specifications. Phase margin 2 35°, Velocity error
constant, 80/sec . using Bode plots. 6

Part B (Modules 111 & IV)

4. a) What are controllability , observability grammians? Explain their

significance. 4

b) Derive Ackermann's formula for the determination of feedback gain. 5
5. a) Explain with suitable examples, how pole cancellation in a system influences its
stability. http:/¢'mxww.ktuonline.com 3
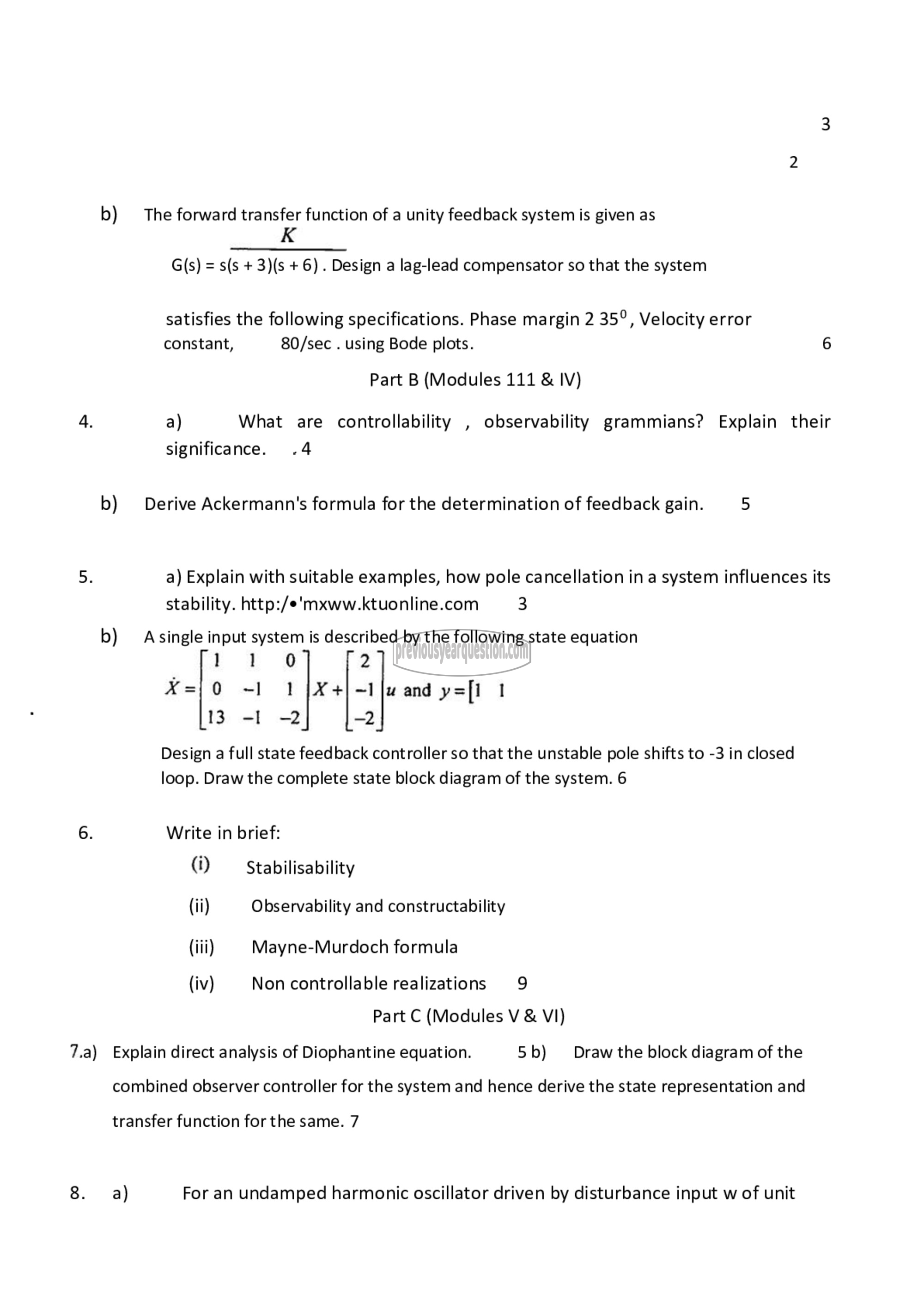
0) Asingle input system is described by the following state equation
1 1 0 2
X=/0 - 1 |ॐ+| 1 |५ 9 += [1 1
13 -[ -2 2

Design a full state feedback controller so that the unstable pole shifts to -3 in closed
loop. Draw the complete state block diagram of the system. 6

6. Write in brief:
)( Stabilisability
(ii) Observability and constructability
(iii) Mayne-Murdoch formula

(iv) Non controllable realizations 9
Part C (Modules V & VI)

7.a) Explain direct analysis of Diophantine equation. 5b) Draw the block diagram of the

combined observer controller for the system and hence derive the state representation and

transfer function for the same. 7

8. 3) For an undamped harmonic oscillator driven by disturbance input w of unit