10 a)
b)
11 a)
b)
12 2
0)
13
14 ஐ
೩)

೧5944 Pages: 3

Derive an expression for the step response of a critically damped second order
system?
Determine the value of gain K and the natural frequency of oscillation “> for

the unity feedback system with forward transfer function 6, (s)= tan)
SS+

which results in a critically damped response when subjected to a unit step
input.
A unity feedback system is characterised by an open loop transfer function

G, (൭ ‏ےت‎
‎»(s) 52 +5545

step input and sketch the response. Evaluate the maximum overshoot and the
corresponding peak time of the system.

. Determine the transient response when subjected to a unit

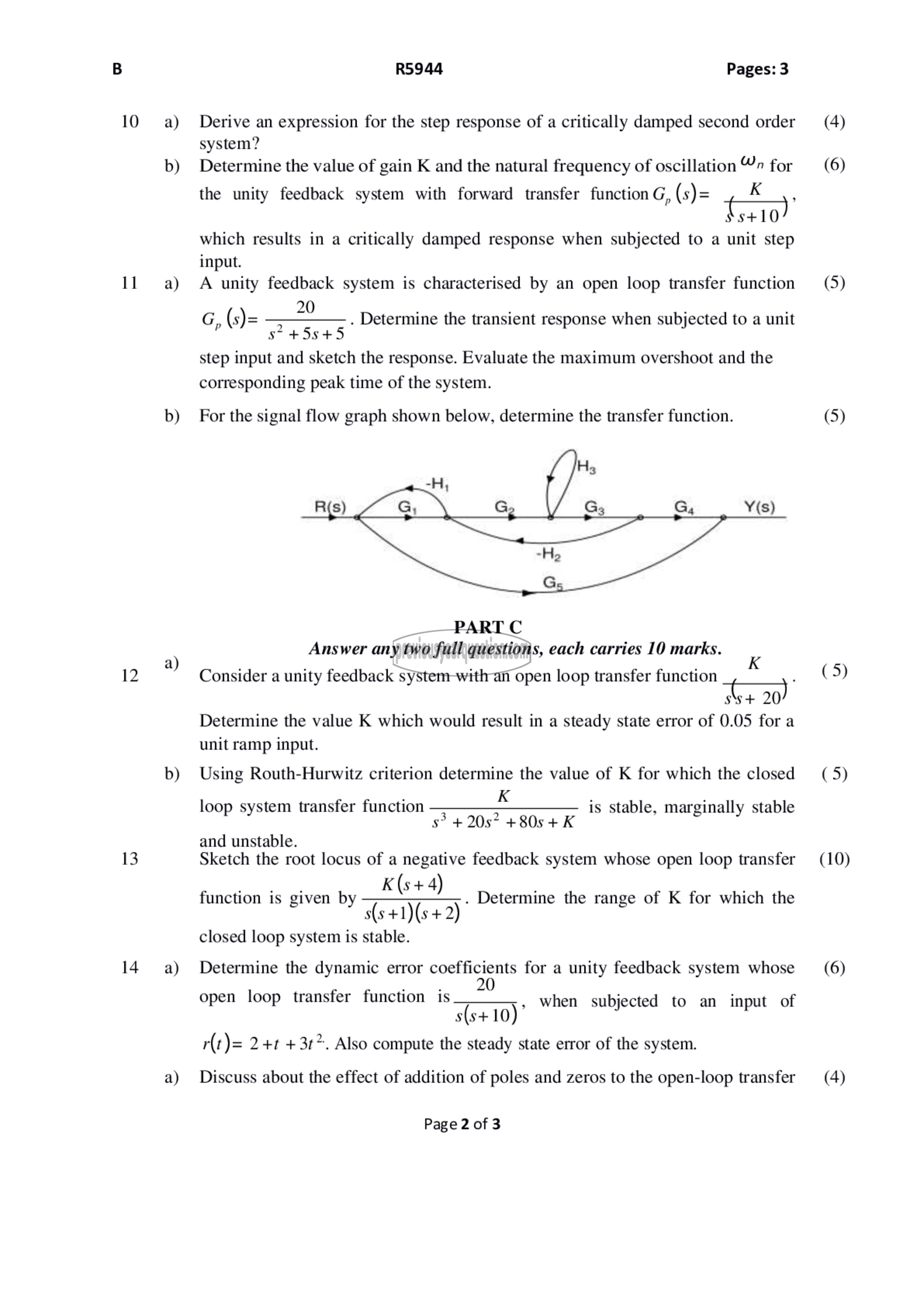
For the signal flow graph shown below, determine the transfer function.

113

-H,

१5)

PART C
Answer any two full questions, each carries 10 marks.

Consider a unity feedback system with an open loop transfer function tray) :
ss+ 0
Determine the value K which would result in a steady state error of 0.05 for a
unit ramp input.
Using Routh-Hurwitz criterion determine the value of K for which the closed
K

loop system transfer function ‏تک‎ ऊर
‏و80 + 2057 + دی‎ + K

is stable, marginally stable

and unstable.

Sketch the root locus of a negative feedback system whose open loop transfer
K(s+4)
s(s +1)(s +2)

closed loop system is stable.

function is given by . Determine the range of K for which the

Determine the dynamic error coefficients for a unity feedback system whose

⋅⋅ 20
open loop transfer function is when subjected to an input of
sist]

r(t)= 241 +312. Also compute the steady state error of the system.

Discuss about the effect of addition of poles and zeros to the open-loop transfer

Page 2 of 3

(4)
(6)

(5)

(5)

(5)

(5)

(10)

(6)

(4)