B4818 Pages: 3

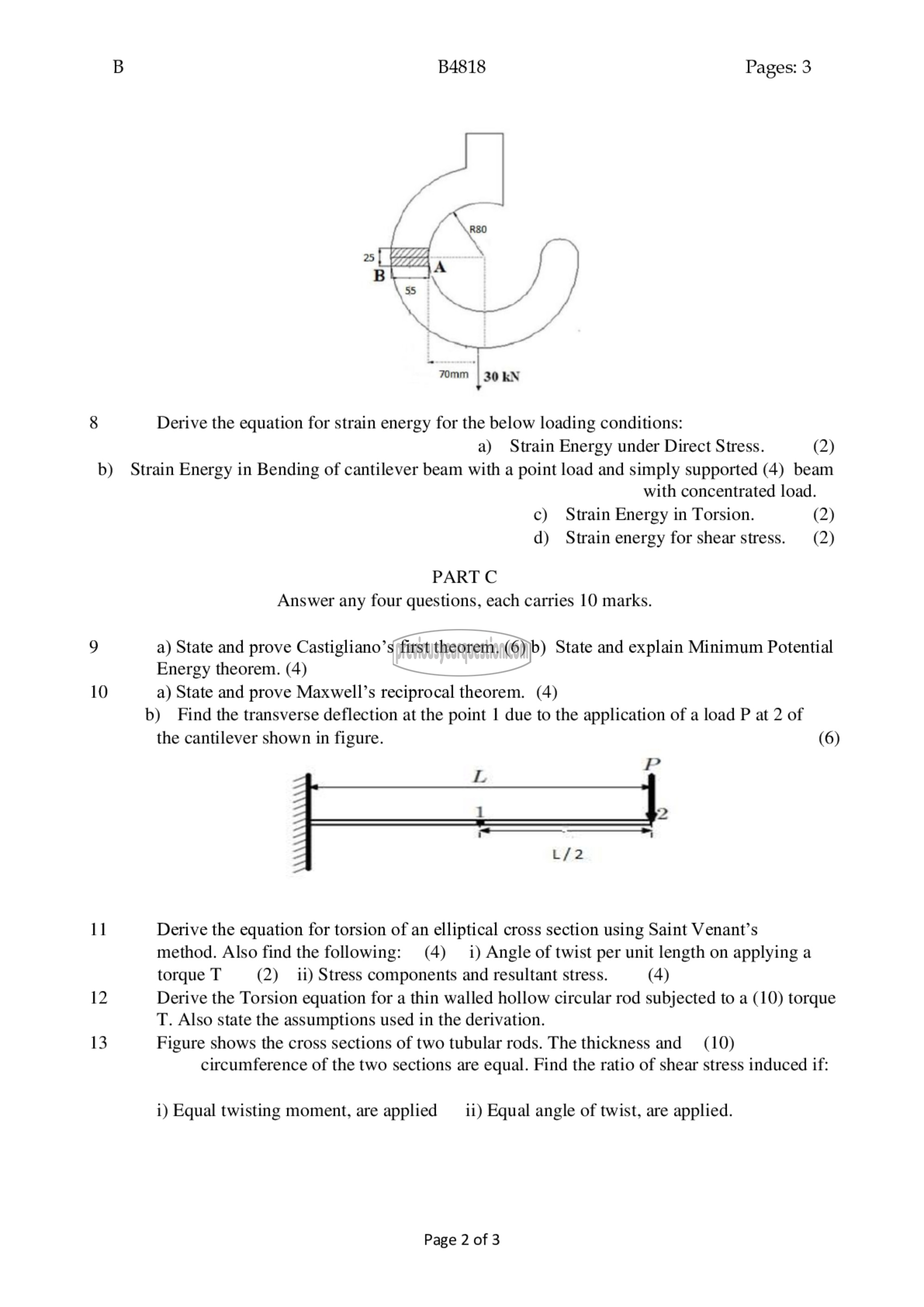
70mm |30 kN

Derive the equation for strain energy for the below loading conditions:
a) Strain Energy under Direct Stress. (2)

b) Strain Energy in Bending of cantilever beam with a point load and simply supported (4) beam

10

1]

12

13

with concentrated load.
c) Strain Energy in Torsion. (2)
d) Strain energy for shear stress. (2)

PART ^
Answer any four questions, each carries 10 marks.

a) State and prove Castigliano’s first theorem. (6) b) State and explain Minimum Potential
Energy theorem. (4)
a) State and prove Maxwell’s reciprocal theorem. (4)

b) Find the transverse deflection at the point | due to the application of a load P at 2 of

the cantilever shown in figure. (6)

Derive the equation for torsion of an elliptical cross section using Saint Venant’s

method. Also find the following: (4) 1) Angle of twist per unit length on applying a

torque T (2) 14) Stress components and resultant stress. (4)

Derive the Torsion equation for a thin walled hollow circular rod subjected to a (10) torque

T. Also state the assumptions used in the derivation.

Figure shows the cross sections of two tubular rods. The thickness and (10)
circumference of the two sections are equal. Find the ratio of shear stress induced if:

i) Equal twisting moment, are applied _ii) Equal angle of twist, are applied.

Page 2 of 3