Pages: 3

6. Consider a thin large rectangular plate, with a small hole at the centre, subjected to uniform
tensile stress from its two ends. Find the variations of stress components around the hole

by Airy’s stress function method. (10)
B B4B142

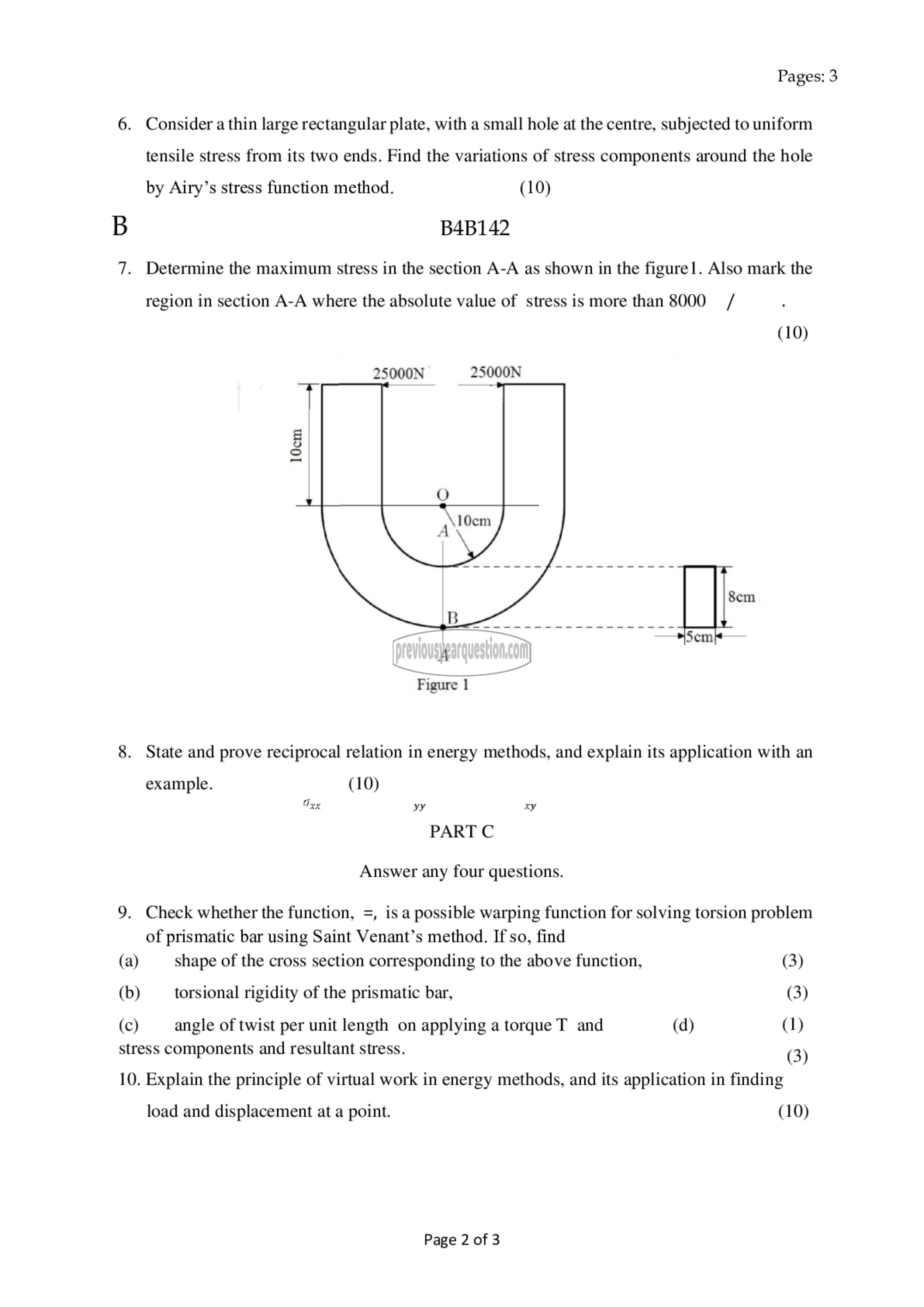
7. Determine the maximum stress in the section A-A as shown in the 180/01. Also mark the

region in section A-A where the absolute value of stress is more than 8000 /
(10)

25000N 25000N

85

A

Figure 1

8. State and prove reciprocal relation in energy methods, and explain its application with an

example. (10)

Oxx yy xy

PART ^
Answer any four questions.

9. Check whether the function, =, is a possible warping function for solving torsion problem
of prismatic bar using Saint Venant’s method. If so, find

(a) shape of the cross section corresponding to the above function, (3)
(b) torsional rigidity of the prismatic bar, (3)
(c) angle of twist per unit length on applying a torque T and (d) (1)
stress components and resultant stress. (3)

10. Explain the principle of virtual work in energy methods, and its application in finding

load and displacement at a point. (10)

Page 2 of 3