C1102 Pages 4

a) Derive the equation of theory of pure bending. (6) b) A rectangular section is to be cut
from a circular log of wood of diameter (4)
500mm. Find the dimensions of strongest section in bending

a) Derive an expression for determining shear stress distribution in a rectangular

(4)

cross-section of width ‘b’ and depth‘d’ and determine the maximum shear stress.

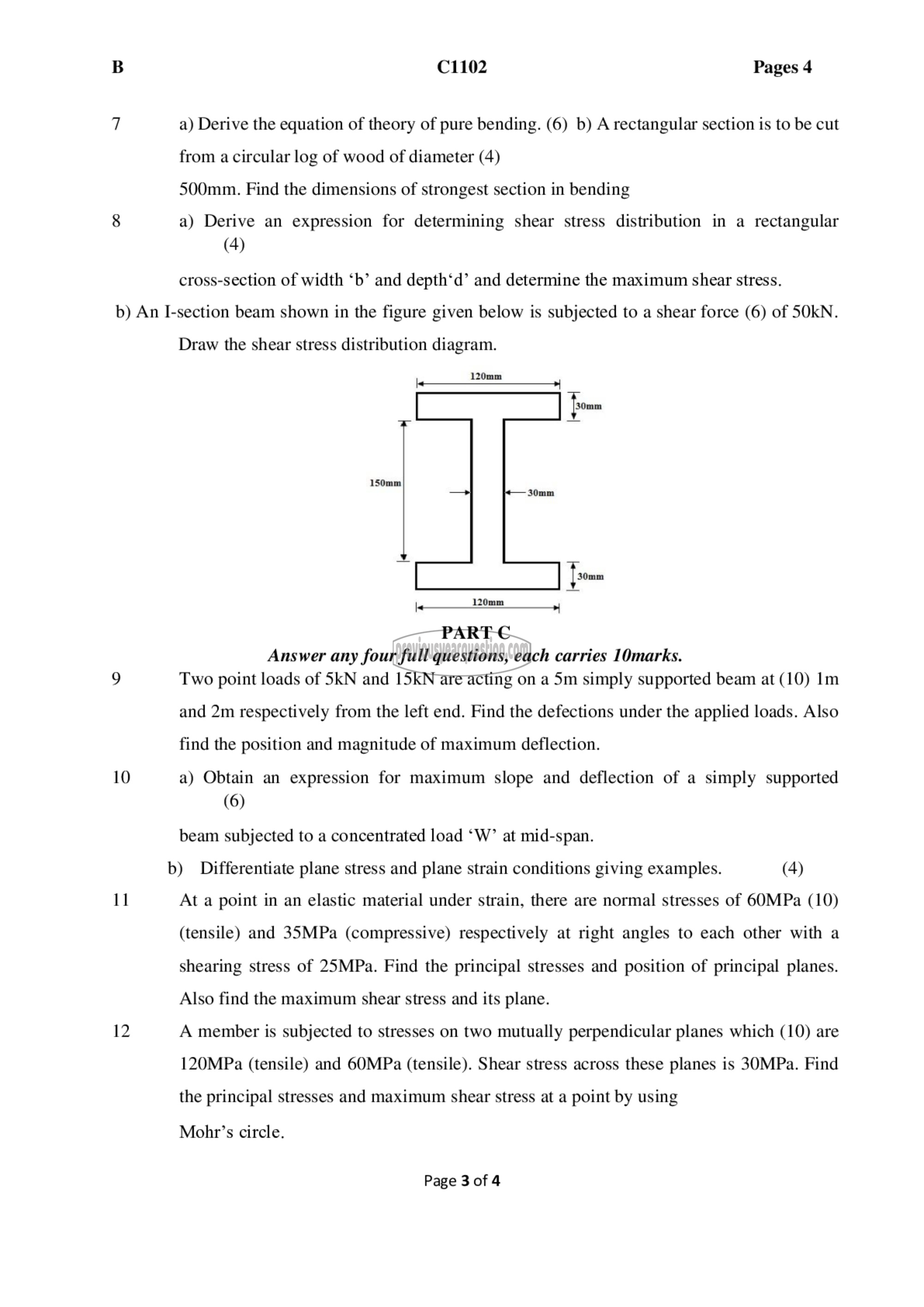
b) An I-section beam shown in the figure given below is subjected to a shear force (6) of SOKN.

10

11

12

Draw the shear stress distribution diagram.

‎பூ‏ سے
‎॥‏
‎150mm‏
‎JE‏
‎॥‏
‎പാഞ്ഞ ച്ച‏
‎PART C‏

‎Answer any four full questions, each carries 10marks.
Two point loads of SKN and 15KN are acting on a 5m simply supported beam at (10) 1൩

‎and 2m respectively from the left end. Find the defections under the applied loads. Also
find the position and magnitude of maximum deflection.

‎a) Obtain an expression for maximum slope and deflection of a simply supported

‎(6)

‎beam subjected to a concentrated load ‘W’ at mid-span.

‎b) Differentiate plane stress and plane strain conditions giving examples. (4)

‎At a point in an elastic material under strain, there are normal stresses of 60MPa (10)
(tensile) and 35MPa (compressive) respectively at right angles to each other with a
shearing stress of 25MPa. Find the principal stresses and position of principal planes.
Also find the maximum shear stress and its plane.

‎A member is subjected to stresses on two mutually perpendicular planes which (10) are
120MPa (tensile) and 60MPa (tensile). Shear stress across these planes is 30MPa. Find
the principal stresses and maximum shear stress at a point by using

‎Mohr’s circle.

‎Page 3 of 4