6 ^] [04
2 ட்‌.
at the level of floor. Find the minimum horizontal force in this condition. ~~~
13 a) Define principal axes and principal moment of inertia. (4)
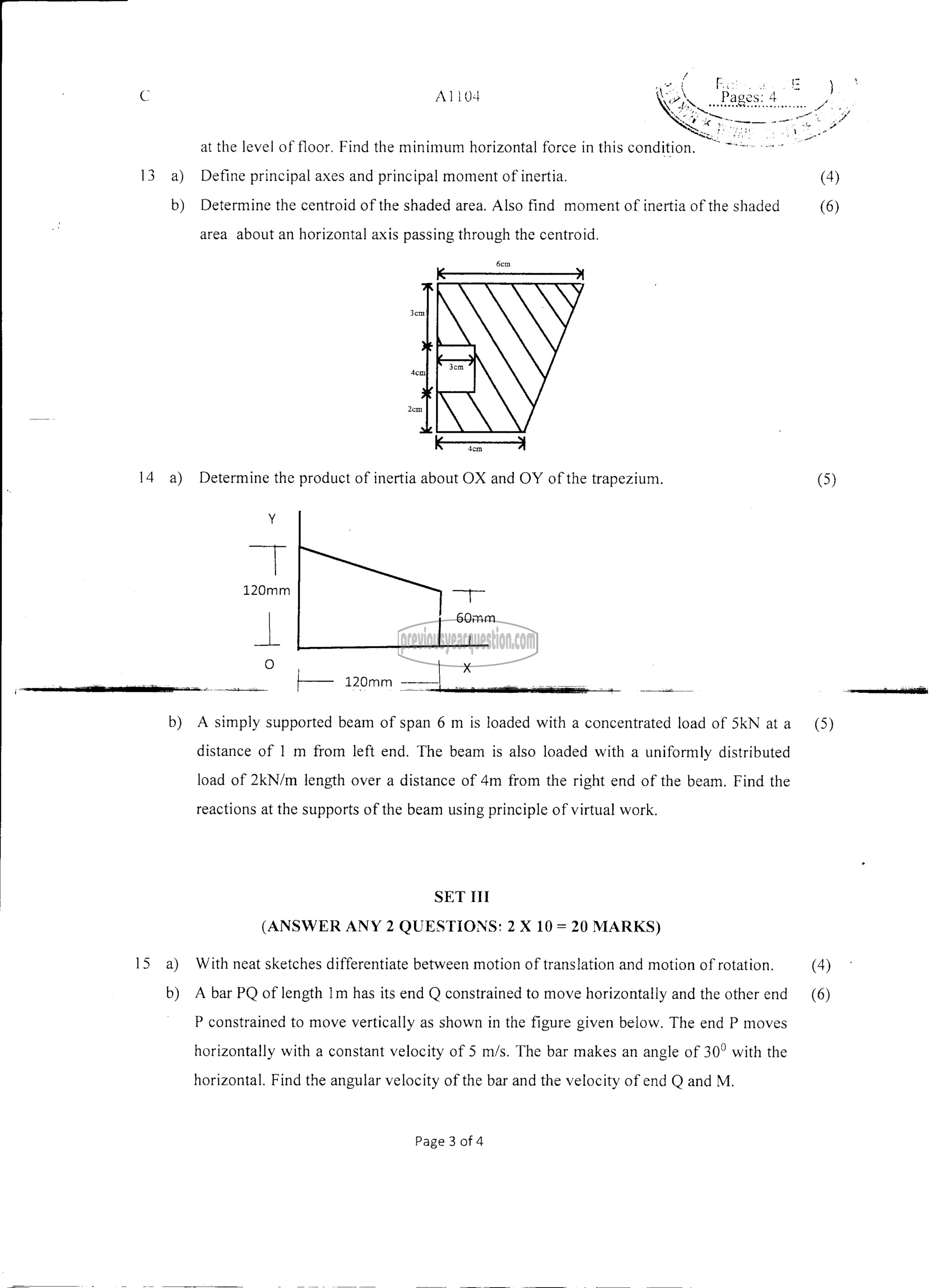
b) Determine the centroid of the shaded area. Also find moment of inertia of the shaded (6)
area about an horizontal axis passing through the centroid.
14 a) Determine the product of inertia about OX and OY of the trapezium. (5)
7
120mm ‏سے‎
‎0 60mm
೦
ಹಾ ‏م‎ 120mm -—— 0
b) A simply supported beam of span 6 m is loaded with a concentrated load of SKN ata (5)
distance of 1 m from left end. The beam is also loaded with a uniformly distributed
load of 2kKN/m length over a distance of 4m from the right end of the beam. Find the
reactions at the supports of the beam using principle of virtual work.
SET 111
(ANSWER ANY 2 QUESTIONS: 2 X 10 = 20 MARKS)
15 8) With neat sketches differentiate between motion of translation and motion of rotation. (4)
b) A bar PQ of length 117 has its end © constrained to move horizontally and the other end (6)

P constrained to move vertically as shown in the figure given below. The end P moves
horizontally with a constant velocity of 5 m/s. The bar makes an angle of 30° with the

horizontal. Find the angular velocity of the bar and the velocity of end Q and M.

Page 3 of 4